Fig. 1 Example of a microwave structure built with several inline rectangular waveguides. Views of the metallic shell (top) and the air volume inside of the structure (bottom)

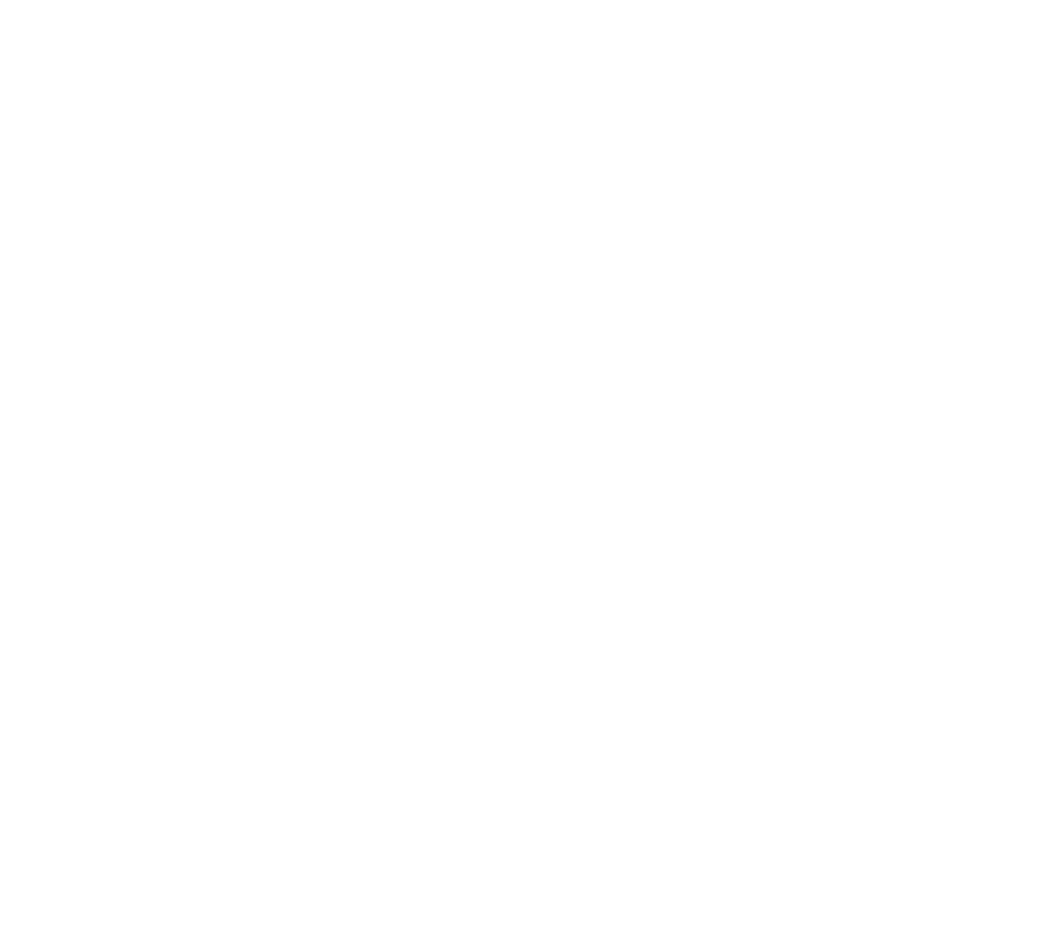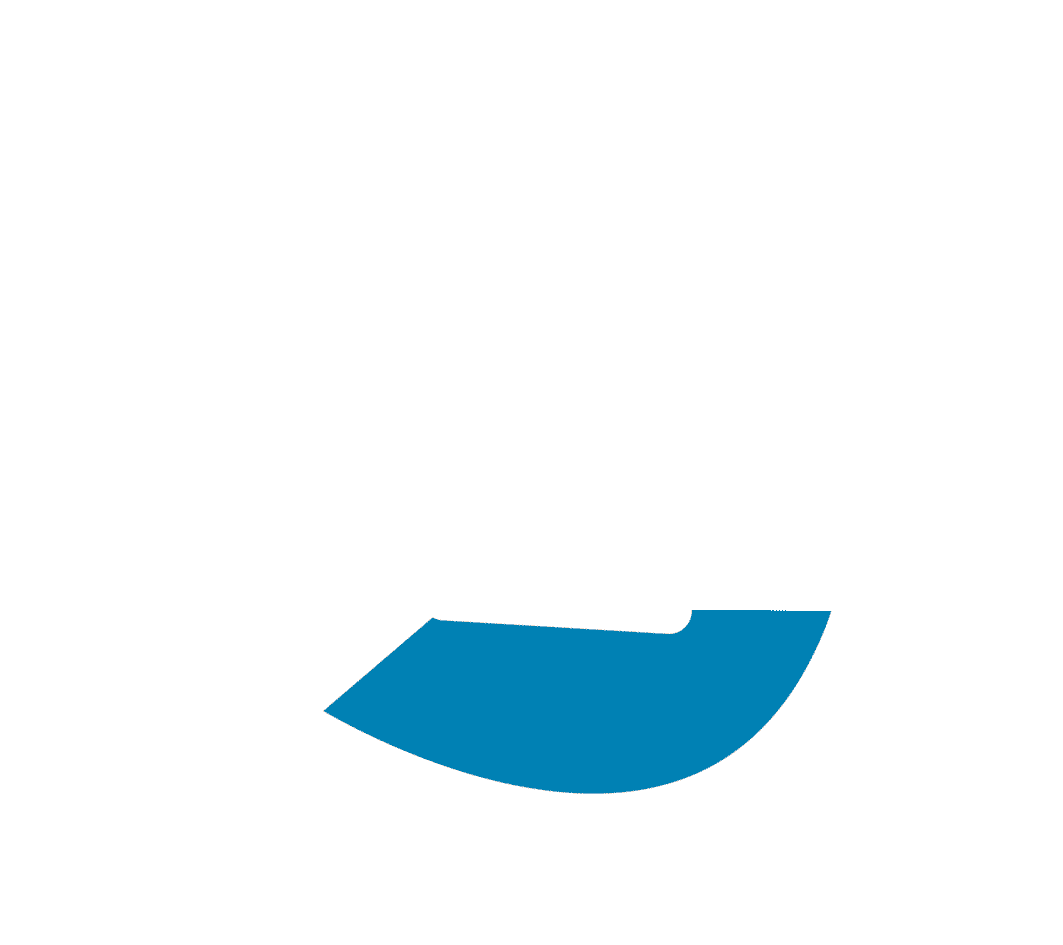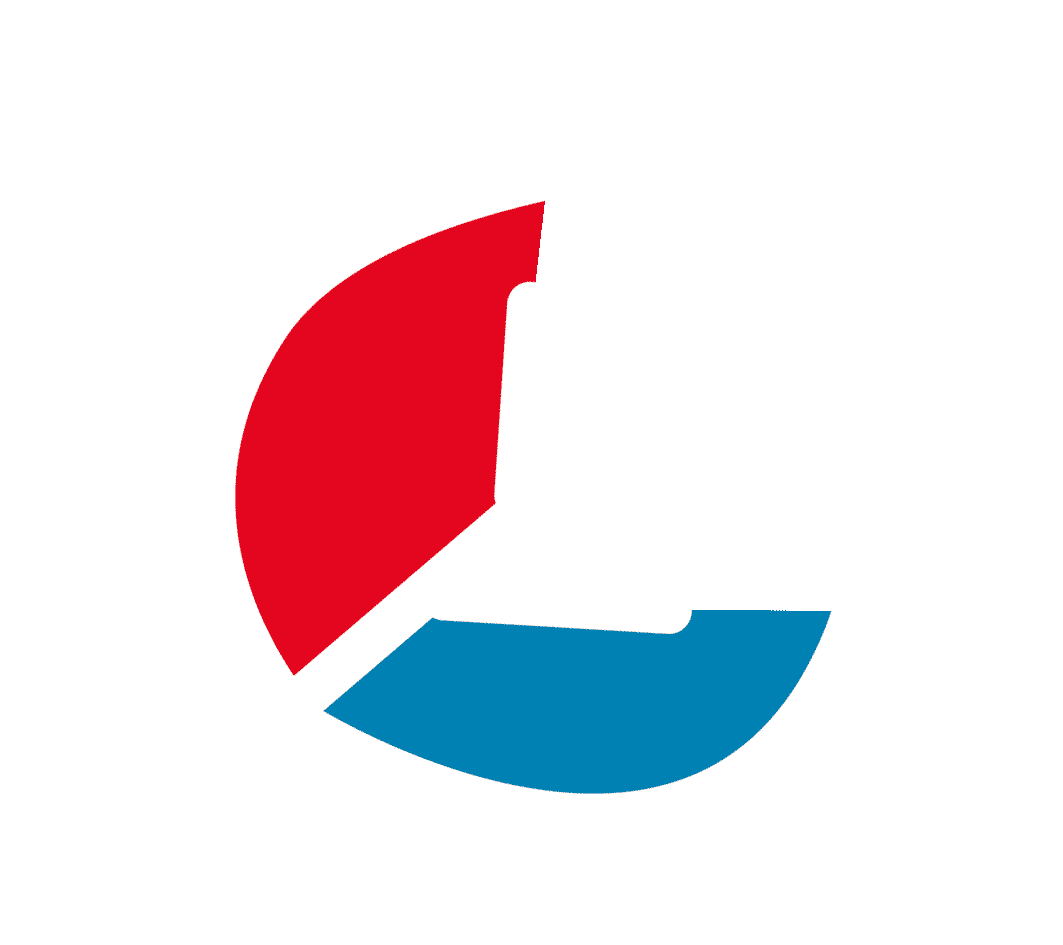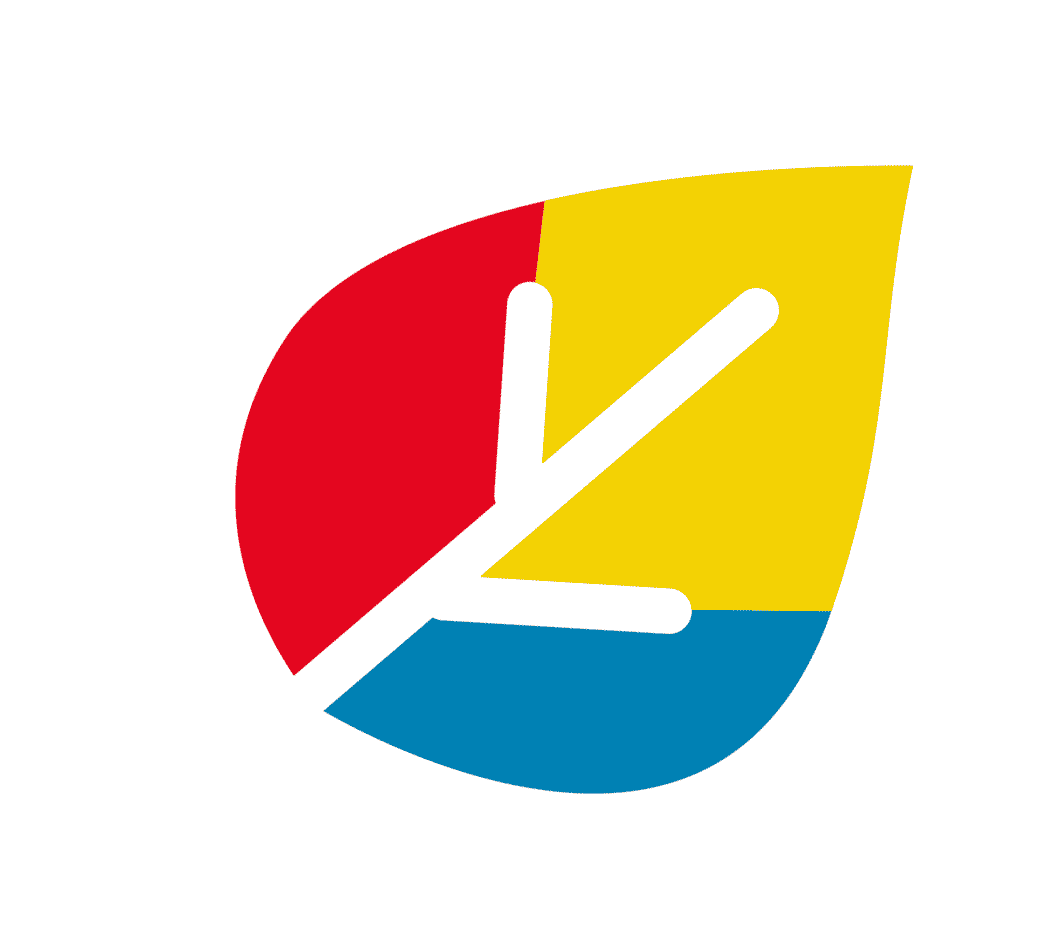
Fig. 2 Building blocks of the previous structure. Each one is characterized by its GSM related modal amplitudes at each side (denoted with a, b). At a final stage, the GSMs are cascaded, providing the system response.
The Mode-Matching Technique
Mode-matching (MM) techniques [1],[2] are among the most efficient and accurate simulation methods to tackle waveguide structures. They have become a standard approach in the resolution of real industrial problems such as waveguide transformers, high performance filters, multiplexers, polarizers, or ortho-mode transducers.
The key idea behind this method consists of the segmentation of the structure under analysis into individual waveguide regions. The electromagnetic (EM) field at each of these regions can be expressed as a weighted superposition of the waveguide modes. The specific amplitude of each mode accounts for the boundary conditions between adjacent regions, as well as for the excitation and load of the whole structure. In this sense, unlike other numerical methods, MM reduces the EM problem to a linear system based on the amplitude of each waveguide mode (scalar complex numbers) rather than the vector fields at each point of a 3D mesh/grid discretization. This creates a considerable reduction in the number of unknowns as well as a more faithful representation of the EM fields, which leads to both a fast and precise resolution of the EM problem.
As an example, the aforementioned approach is implemented as follows in the structure of Fig.1. First, the structure under analysis is segmented into building blocks. These are typically discontinuities between waveguides and more complex junctions involving several waveguide ports. These building-blocks are then further divided into waveguide regions, where the EM field is theoretically expanded in the formulation of the algorithm as an infinite series of propagating and evanescent modes. For computational reasons, these series are truncated according to the degree of accuracy required. This is determined by the relative number of modes retained in each field expansion and the total number of modes used in the entire problem.
At the interface between adjacent regions, the modal series defined at each side must match to fulfill boundary conditions. This leads to a linear system, whose solution determines the amplitude of the modes at each waveguide region. This is usually formulated using a scattering matrix formalism, although other approaches based on admittance or impedance matrices are also possible. Thus, the characterization of each building block results in the Generalized Scattering Matrix (GSM), which includes both propagating and evanescent modes.
Finally, the GSM of each building block is cascaded, providing the response of the entire structure. In this sense, the initial problem has been reduced to a multi-port circuit problem (one circuit port per mode), capturing all the EM interactions within the structure at the same time. The S-parameters of the modes at the input/output ports can be extracted from the final GSM.
Moreover, depending on the application, the analysis may require the computation of the EM fields at any point on the structure. In that case, the fields can be reconstructed by summing the modal series previously computed at each specific waveguide region. The main advantage of this approach is that it does not require any additional interpolation procedure since the waveguide modes are known in an analytical or quasi-analytical form.
The current capabilities of the MM solver in SEMCAD X allow for the simulation of structures composed of inline waveguide sections with rectangular, circular, elliptical and circular/elliptical coaxial sections. In addition, structures with N-furcations and cubic-junctions can also be tackled following a similar approach. The combination of these components provides a simulation toolset capable of efficiently and accurately solving a wide variety of waveguide problems.
References
[1] A. Wexler, “Solution of waveguides discontinuities by modal analysis,” IEEE Transactions on Microwave Theory and Techniques, vol. 15, pp. 508–517, 1967.
[2] H. Patzelt and F. Arndt, “Double-plane steps in rectangular waveguides and their application for transformers, irises, and filters”, IEEE Transactions on Microwave Theory and Techniques, vol. 82, no. 5, pp. 771–776, May 1982.